Angular Momentum: Definition, Equation, Units (W/ Diagrams & Examples)

Consider the scene: You and a friend, owing to issues beyond your control, are standing at the top of a long, downward-sloping ramp. Each of you has been given a ball exactly 1 m in radius. You've been told that yours is made of a uniform, foam-like material and has a mass of 5 kg. Your friend's ball also has a mass of 5 kg, which you verify with a handy scale.

Your friend wants to bet you that if you release the two balls at the same time, yours will get to the bottom first. You're tempted to argue that since the balls have the same mass and the same radius (and hence volume), they will be accelerated by gravity down the ramp to the same velocity throughout the descent. But something stops your betting "momentum," and you don't take the wager....

...wisely, as it turns out. Though it makes no sense at first, your friend's ball, by all appearances a twin of your own, moves down the ramp more slowly than yours does. After the experiment is over, you demand the balls be dismantled and examined for signs of trickery. Instead, all you find is that the 5 kg of mass in your friend's ball was confined to a thin shell around the outside, with the interior hollow.

What about the above-described configuration tilts the value of v in your ball's favor? As happens, just as ​forces​ change the ​linear momentum​ of objects with ​linear velocity​, ​torques​ change the ​angular momentum​ of objects with ​angular velocity​.

A rigid rolling object has both linear momentum and angular momentum, because as its center of mass moves with a constant velocity v (equal to the tangential velocity of the ball or wheel), every other portion of the object rotates about that center of mass with angular velocity ω.

How mass is distributed within an object has no bearing on its linear momentum, but determines its angular momentum exquisitely. It does this through a "mass-like" (for rotational purposes) quantity called the moment of inertia, higher values of which imply both more difficulty in getting something rotating and more difficulty stopping it once it's already rotating.

Angular momentum is a measure of how difficult it is to change an object's rotational motion. It depends on the object's moment of inertia and its angular velocity. Angular momentum is a conserved quantity, meaning that the sum of the angular momenta of the particles in a closed system is always the same, even as that of individual particles can fluctuate.

Angular momentum is, as noted, also a function of the distribution of mass about an axis. To gain an intuitive sense of this, imagine standing 1 foot from the center of an enormous merry-go-round that makes one revolution every 10 seconds. Now imagine being on the same contraption with the same angular velocity while standing 1 ​mile​ from the center. It doesn't take much imagination to conceive of the difference in angular momentum in these two scenarios.

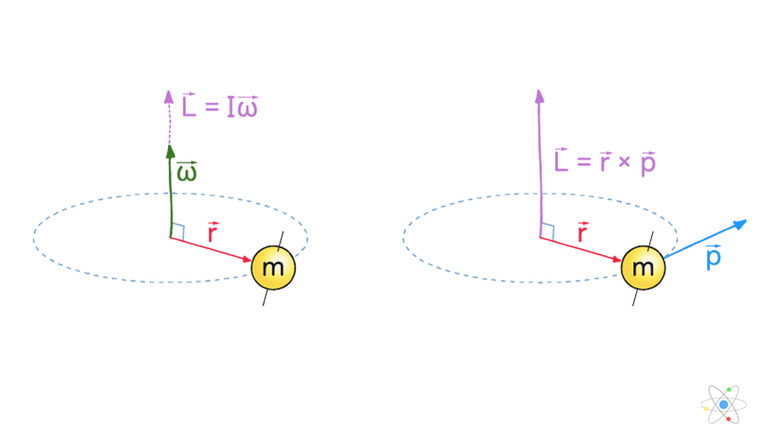
​**Angular momentum is the product of the moment of inertia times its angular velocity, or:
**​

\(L=I\omega\)

where ​L​ = angular momentum in kg∙m²/s, ​I​ = moment of inertia in kg∙m², and ω = angular velocity in radians per second (rad/s).

Dana Chen | Sciencing

•​I​ is also called the second moment of area.

Note that the discussion has broadened from a point mass to a solid body, such as a cylinder or sphere, rotating about an axis. The center of mass of an object is often not at its ​geometric​ center, so values of ​I​ depend on how the mass of the object is distributed. Often this is symmetrical but not uniform, such as a hollow disk with all its mass in a thin strip on the outside (in other words, a ring).

The angular momentum vector points along the axis of rotation, perpendicular to the plane formed by ​r​, the circular "sweep" of any point in the object through space.

A reference chart for the value of ​I​ for different common shapes is found in the Resources. Use these to get started on a few basic angular momentum problems.

•Note that ​I​ for a spherical shell is (2/3)mr² while that of a sphere is (2/5)mr².  Going back to the wager in the introduction, you can now see that your friend's ball has (2/3)/(2/5) = 1.67 times the moment of inertia as your own, explaining yours winning the "race."

1. A disk with rotational inertia ​I​ of 1.5 kg∙m²/s rotates about an axis with an angular velocity ​ω​ of 8 rad/s. What its its angular momentum ​L​? 

\(L=I\omega=(1.5)(8)=12\text{ kgm}^2\text{/s}\)

2\. A thin rod 15 m long with a mass of 5 kg – the hand of a massive clock, say – rotates about a point fixed at one end with an angular velocity ​ω​ of 2π rad/60 s = (π/30) rad/s. What is its angular momentum ​L​?

This time, you need to look up the value of ​I​. For a thin rod moving in this manner, ​I​ = (1/3)m​**r²**​.

\(L=I\omega=\frac{1}{3}(5)(15)^2(\pi /30)=\frac{375\pi}{30}=39.3\text{ kgm}^2\text{/s}\)

Compare this to the answer in the first example. Does this surprise you? Why or why not?

"Conservation" means something a little different in physics than it does in the realm of ecosystems. It simply means that the total amount of conserved quantities (energy, momentum, mass and inertia are the "big four" conserved quantities in physics) in a system, including the universe, always stays the same. If you attempt to "eliminate" energy, it simply shows up in another form, and any attempt to "create" it relies on a pre-existing source.

The law of conservation of angular momentum states that in a closed system, the total angular momentum cannot change. Because angular momentum depends on angular velocity and moment of inertia, one can predict how either of these quantities must then change in relation to one another in a given situation.

•Formally, since torque can be expressed as ​τ​ = d​L​/dt (the rate of change if angular momentum with time), when the sum of the torques in a system is zero, then d​L​/dt must be zero as well and there is no change in angular momentum in the system over the time frame in which the system is assessed. Conversely, if L is not constant, this implies an imbalance of torques in the system (i.e, ​**τ_net**​ is ​not​ equal to zero).

This is an important concept in many mechanics examples from daily life. A classic example is the ice skater: When she jumps in the air to do a triple axel, she pulls her limbs in tightly. This decreases her overall radius around her axis of rotation, changing her distribution of mass so that her moment of inertia decreases (remember, ​I​ is proportional to m​**r²**​).

Because angular momentum is conserved, however, if ​I​ decreases, her angular velocity must increase; this is how she spins quick enough to complete several rotations midair! When she lands, she does the reverse – she spreads out her limbs, changing her mass distribution to increase her moment of inertia, slowing down her rotation rate (angular velocity) in turn.

All throughout, the angular momentum of the system is constant, but the variables that determine the magnitude of the angular momentum can be manipulated, and to strategic effect, as in this case.

Starting in the 1600s, Isaac Newton set about effectively revolutionizing mathematical physics. Having co-invented calculus, he was well positioned to make formal assertions about the presumably universal laws governing the motion of objects, both translational (linearly and through space) and rotationally (cyclically and about an axis).

•The various ​conservation laws​ that receive ample mention later are not Newton's brainchildren, but significant relationships exist between these and the laws of motion.

​Newton's first law​ states that an object at rest or moving with constant velocity will remain in this state unless an outside force acts on the object. This is also called the ​law of inertia.​

​Newton's second law​ asserts that a net force ​_F_net​ acts on a particle with mass ​m_​, it will tend to change the velocity of, or accelerate, that mass. This famous relationship is expressed mathematically as ​**F_net​ = m​a**​.

​Newton's third law​ says that for every force that exists in nature, there exists a force equal in magnitude but pointing in exactly the opposite direction. This law has important implications for conserved properties of motion, including angular momentum.

Now is an excellent time to review the nature, rules and relationships between ​force​, ​momentum​ (mass times velocity) and ​energy​, which inform not only discussions about angular momentum but everything else in classical physics.

As noted, unless an object experiences an external force (or in the case of a rotating object, external torque), its motion continues unaffected. On Earth, however, gravity is virtually always in the mix, as are the lesser contributors air drag and various kinds of frictional forces, so nothing simply keeps moving unless it is occasionally given energy to replace what is "taken" by these chronic "motion thieves."

To simplify, a particle has a ​total energy​ consisting of ​internal energy​ (e.g., the vibration of its molecules) and ​mechanical energy​. Mechanical energy is turn the sum of ​potential energy​ (PE; "stored" energy, usually via gravity) and ​kinetic energy​ (KE; energy of motion). Helpfully, PE + KE + IE= a constant for all systems, be it a point mass (single particle) or a variety of whizzing, interacting masses.

When you hear terms related to motion, such as velocity, acceleration, displacement and momentum, you probably assume by default that the context is linear motion. Rotational motion, in fact, has its own unique but analogous quantities.

Whereas linear displacement is measured in meters (m) in SI units, angular displacement is measured in radians (2π rad = 360 degrees). Accordingly, ​angular velocity​ is measured in rad/s and is represented by ​ω​, the Greek letter omega.

However, as a point mass moves around its axis of rotation, in addition to angular velocity, the particle is tracing out a circular path at a given rate, akin to linear motion. This rate is the ​tangential velocity​ ​**v_t**​​,​ and is equal to r​ω,​ where ​r​ is the radius, or distance from the axis of rotation.

Relatedly, ​angular acceleration​ ​α​ (Greek alpha) is the rate of change of angular velocity ​ω​ and is measured in rad/s². There is also a ​centripetal acceleration​ ​**a_c​ given by ​v_t²/r,**​ which is directed inward toward the axis of rotation.

•While discussion of angular momentum, the counterpart of m​v​ in linear terms, will be given thorough discussion soon, know that one of its components, ​I​, can be thought of as a rotational analogue of mass. 

Angular momentum, like force, displacement, velocity and acceleration, is a ​vector quantity​, because such variables include both a ​magnitude​ (i.e., a number) and a ​direction​, often given terms of its individual x-, y- and z-components. Quantities that only contain a numerical element, such as mass, time, energy and work, are known as ​scalar quantities​.