How To Calculate Hypotenuse

The hypotenuse is one of many terms in math and science that most people seem to have heard, but few can define or describe properly. It refers to the longest side of a right triangle, which is a type of geometric construct with very basic requirements but a practically unlimited range of sizes and overall shapes.

A right triangle is a triangle with an angle of 90 degrees. This sole requirement results in triangles possessing a marvelous array of unique mathematical properties, including ways to determine the length of the hypotenuse given information about the other two sides or one side and one of the two non-90-degree angles.

The hypotenuse of a right triangle is the longest side, which always lies across from the right angle. The lengths of the other two sides, called legs, can vary almost infinitely because the other two angles can each be between just over 0 degrees and just under 90 degrees provided their sum is 90. This follows from that fact that the sum of the angles of any triangle is 180 degrees and a right angle is 90 degrees.

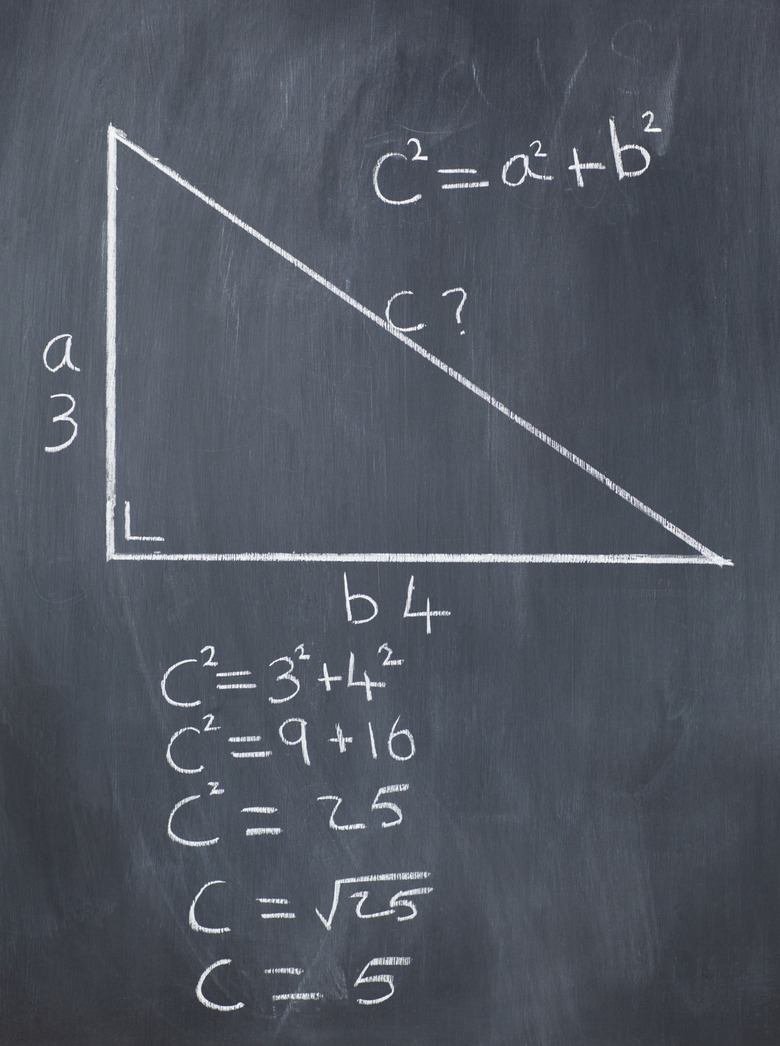
The hypotenuse formula, which you may already know, is the formal mathematical expression of the Pythagorean theorem. It asserts that the sum of the squares of the lengths of the shorter two sides of the triangle a and b is equal to the square of the length of the hypotenuse c:

\(a^2 + b^2 = c^2\)

You can see from the formula for the Pythagorean theorem that taking the square root of each side gives an explicit formula for the value of the hypotenuse:

\(c = \sqrt{a^2 + b^2}\)

If you have the values for the lengths of both legs of the triangle, you do not need any information about the magnitude of the angles to figure out the length of the hypotenuse. All you need to do is square each leg value independently, add the results together, and take the square root of this sum to get the answer.

• Do not make the mistake of adding the values of the legs first and then squaring the result, or your answer will be incorrect.

The hypotenuse equation above is only of use is you know the length of both legs. In some situations, you may be given the length of only one leg along with the magnitude of one of the two non-right angles. This angle might be adjacent to the known leg, or it may be across from it (refer to a diagram for a better understanding of this).

In a properly labeled right triangle, side a lies between angle B and the right angle C, and side b lies between angle A and C; the hypotenuse c thus joins A and B. This gives rise to the following trigonometric relationships:

sin A = a/c, sin B = b/c
cos A = b/c, cos B = a/c
tan A = a/b, tan B = b/a

Which relationships you use depends on what angle and what side you know. For reference, the sine of an angle is the value of the opposite side divided by that of the hypotenuse; the cosine is the value of the adjacent side divided by that of the hypotenuse; and the tangent is the value of the opposite side divided by that of the adjacent side.

For example, if the side a = 15, and the angle A = 55 degrees, you can use the sine function on your calculator to find the hypotenuse. Since sin A = a/c, you have c = a/sin A = 15/sin 55. This turns out to be 15/ 0.8192 = 18.31.