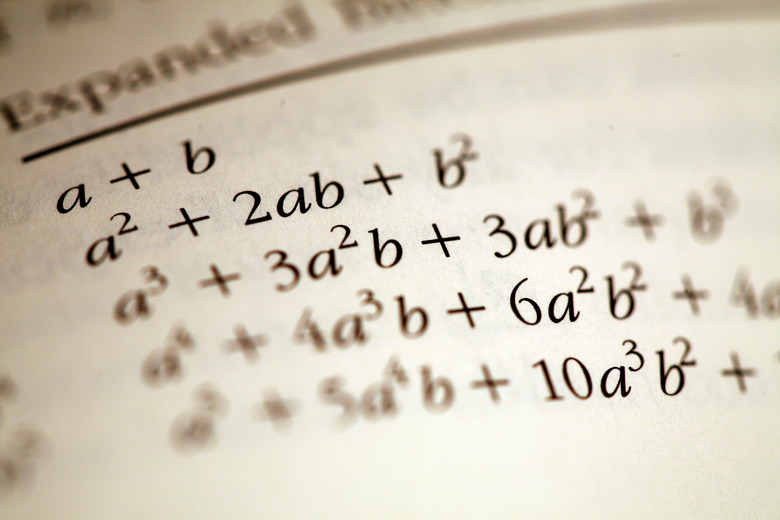How To Cube Binomials

Algebra is full of repeating patterns that you could work out by arithmetic every time. But because those patterns are so common, there's usually a formula of some sort to help make the calculations easier. The cube of a binomial is a great example: If you had to work it out every time, you'd spend a lot of time toiling over pencil and paper. But once you know the formula for solving that cube (and a few handy tricks for remembering it), finding your answer is as simple as plugging the right terms into the right variable slots.

There's no need to panic when you see a problem like (a + b)³ in front of you. Once you break it down into its familiar components, it'll start to look like more familiar math problems you've done before.

In this case, it helps to remember that

**(a + b)³**

is the same as

(a + b)(a + b)(a + b), which should look a lot more familiar.

But instead of working out the math from scratch every time, you can use the "shortcut" of a formula that represents the answer you'll get. Here's the formula for the cube of a binomial:

**(a + b)³ = a³ + 3a²b + 3ab² + b³**

To use the formula, identify which numbers (or variables) occupy the slots for "a" and "b" on the left side of the equation, then substitute those same numbers (or variables) into the "a" and "b" slots on the right side of the formula.

Example 1: Solve **(x + 5)³**

As you can see, x occupies the "a" slot in the left side of your formula, and 5 occupies the "b" slot. Substituting x and 5 into the right side of the formula gives you:

**x³ + 3x²5 + 3x5² + 5³**

A little simplifying gets you closer to an answer:

**x³ + 3(5)x² + 3(25)x + 125**

And finally, once you've simplified as much as you can:

**x³ + 15x² + 75x + 125**

You don't need a different formula to solve a problem like **(y – 3)³. If you recall that y – 3 is the same as y + (-3), you can simply rewrite the problem to [y + (-3)]³** and solve it using your familiar formula.

Example 2: Solve **(y – 3)³**

As already discussed, your first step is to rewrite the problem to **[y + (-3)]³**.

Next, remember your formula for the cube of a binomial:

**(a + b)³ = a³ + 3a²b + 3ab² + b³**

In your problem, y occupies the "a" slot on the left side of the equation, and -3 occupies the "b" slot. Substitute those into the appropriate slots on the right side of the equation, taking great care with your parentheses to preserve the negative sign in front of -3. This gives you:

**y³ + 3y²(-3) + 3y(-3)² + (-3)³**

Now it's time to simplify. Again, pay close attention to that negative sign when you apply exponents:

**y³ + 3(-3)y² + 3(9)y + (-27)**

One more round of simplifying gives you your answer:

**y³ – 9y² + 27y – 27**

Always pay close attention to where the exponents are in your problem. If you see a problem in the form **(a + b)³, or [a + (-b)]³, then the formula being discussed here is appropriate. But if your problem looks like (a³ + b³) or (a³ – b³)**, it's not the cube of a binomial. It's the sum of cubes (in the first case) or the difference of cubes (in the second case), in which case you apply one of the following formulas:

**(a³ + b³) = (a + b)(a² – ab + b²)**

**(a³ – b³) = (a – b)(a² + ab + b²)**