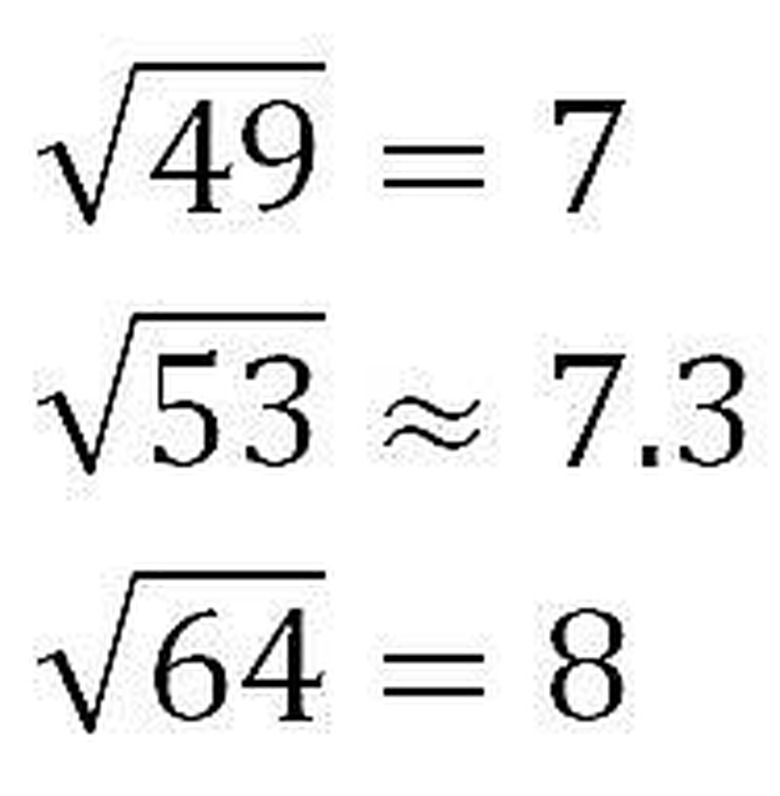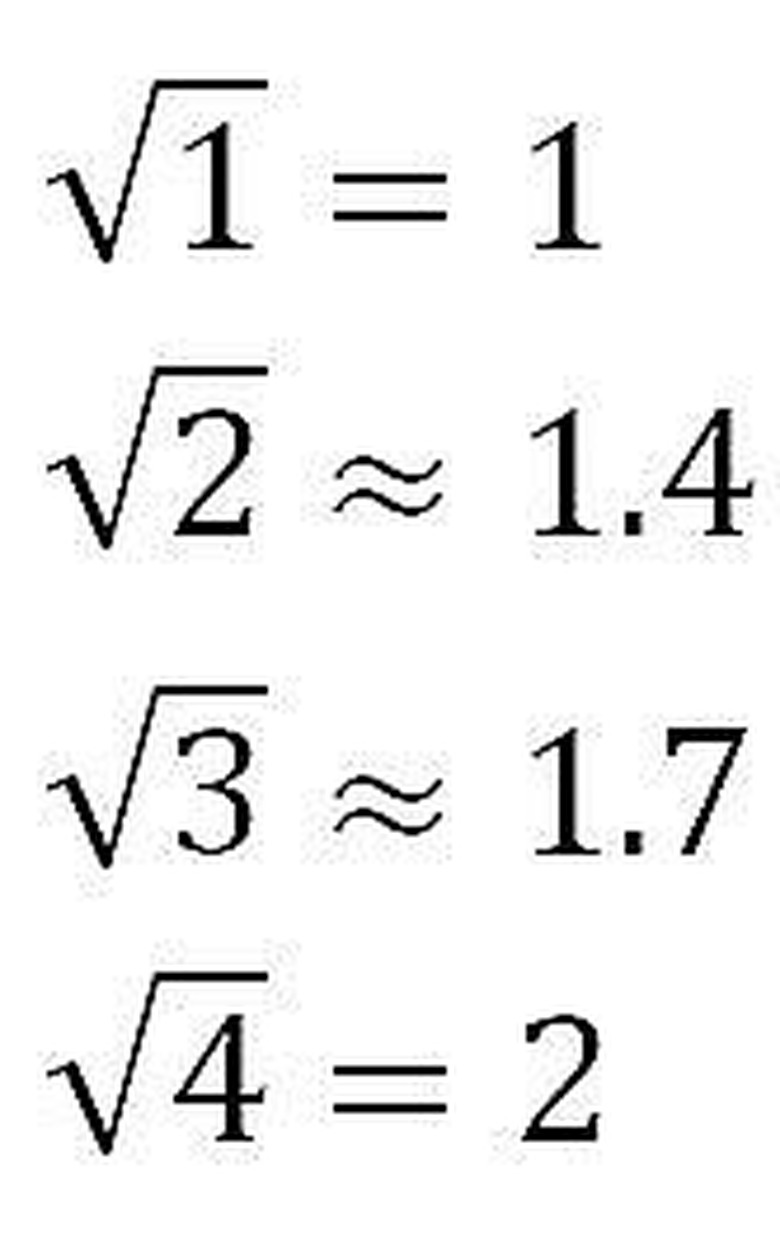How To Estimate Square Roots (Radicals)

In math, it is sometimes important for us to be able to estimate the values of square roots (radicals). This is especially the case on exams which do not permit the use of a calculator, and you are trying to eliminate wrong answers, or check the reasonableness of your answer. Also, in geometry, the values sqrt(2) and sqrt(3) come up so frequently that it is essential to know their approximate values.

This article shows you the steps to estimate a square root. The article assumes that you have a basic understanding of square roots and perfect squares. See the Reference section for more information.

Step 1

To estimate the value of the square root of a number, find the perfect squares are above and below the number. For example, to estimate sqrt(6), note that 6 is between the perfect squares 4 and 9. Sqrt(4) = 2, and sqrt(9) = 3. Since 6 is closer to 4 than it is to 9, we'd expect its square root to be closer to 2 than it is to 3. It's actually about 2.4, but as long you knew it was in that ballpark, you'd be fine. Even just knowing that it was somewhere between 2 and 3 would be to your advantage.

Step 2

Let's try another example. Estimate sqrt(53). 53 is between the perfect squares 49 and 64, the square roots of which are 7 and 8, respectively. 53 is closer to 49 than to 64, so it would be reasonable to estimate sqrt(53) to be between 7 and 7.5. It turns out that it's about 7.3.

Step 3

There are two square roots that come up very frequently in geometry. They are sqrt(2) and sqrt(3). It is very important that you memorize their approximate values. Note that sqrt(1) is 1, and sqrt(4) is 2. Based on this, it should come as no surprise that sqrt(2) is approximately 1.4, and sqrt(3) is approximately 1.7.

The most important thing is to remember that sqrt(2) is greater than 1, and sqrt(3) is less than 2. Another article discusses the application of these square roots in working with right triangles and the Pythagorean Theorem.

Step 4

Students should make sure that they are comfortable with estimating square roots, and for that matter estimating all of their answers to see if they are reasonable. This will usually allow you to catch your mistakes before you hand in your exams.