A hyperbola is a type of conic section formed when both halves of a circular conical surface are sliced by a plane. The common set of points for these two geometric figures form a set. The set is all points "D," so that the difference between the distance from "D" to the foci "A" and "B" are a positive constant "C." The foci are two fixed points. On the Cartesian plane, the hyperbola is a curve that can be expressed by an equation that can’t be factored into two polynomials of a lesser degree.
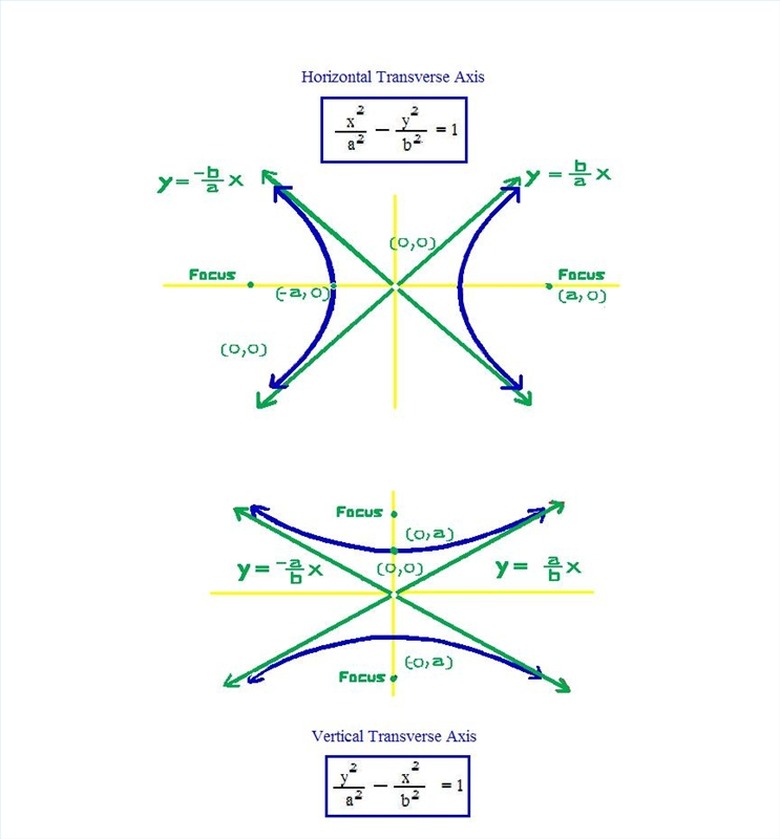
Solve a hyperbola by finding the x and y intercepts, the coordinates of the foci, and drawing the graph of the equation. Parts of a hyperbola with equations shown in picture: The foci are two points determine the shape of the hyperbola: all of the points "D" so that the distance between them and the two foci are equal; transverse axis is where the two foci are located; asymptotes are lines showing the slope of the arms of the hyperbola. The asymptotes get close to the hyperbola without touching it.
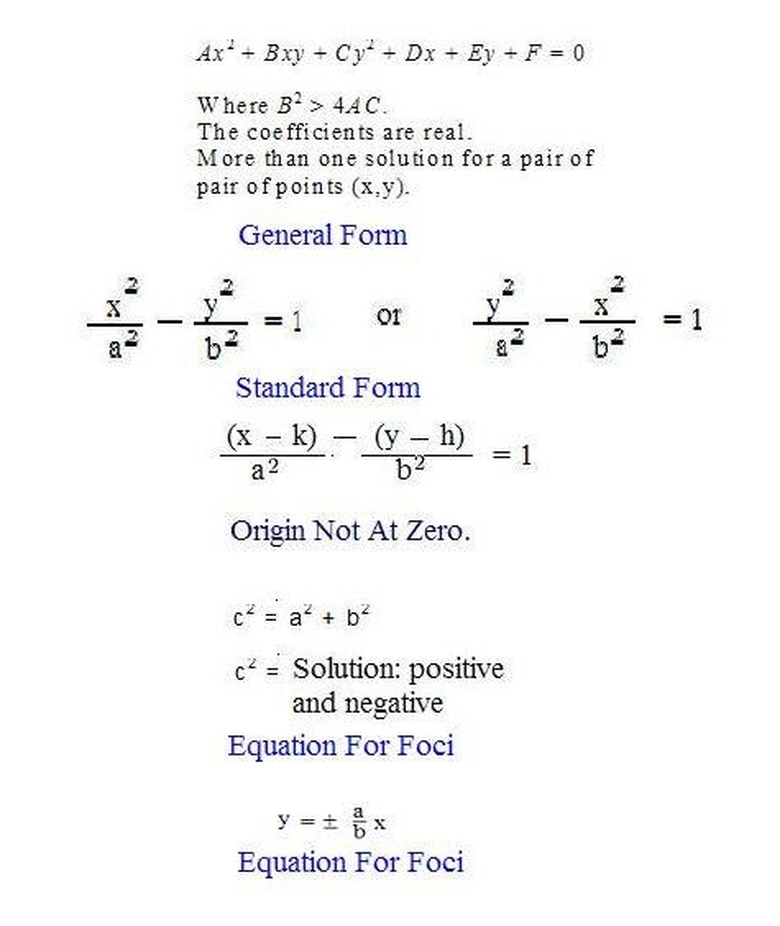
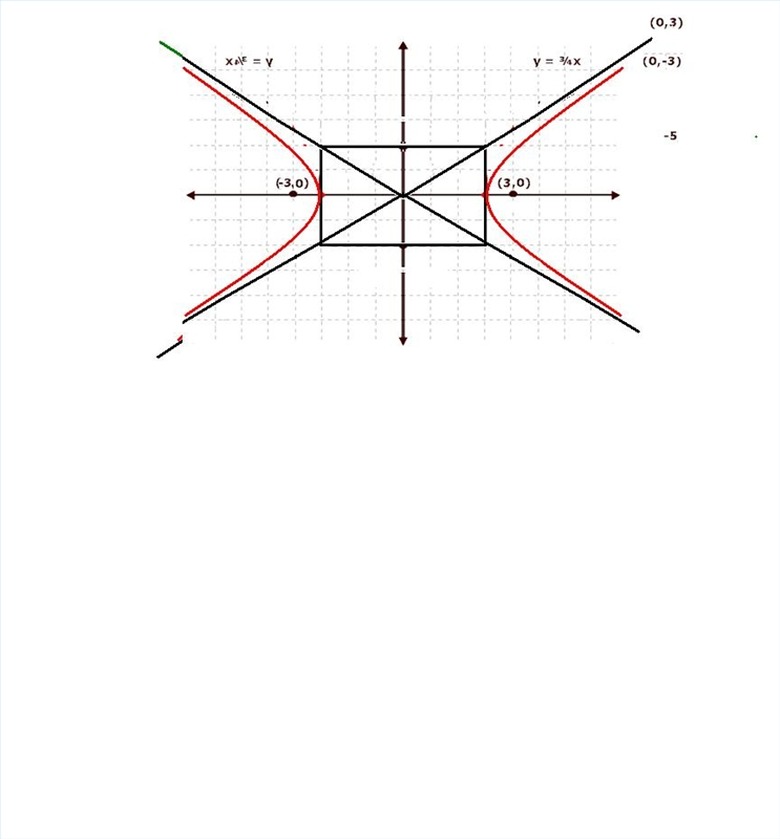
Set up a given equation in the standard form that is shown in the picture.Find the x and y intercepts:Divide both sides of the equation by the number on the right side of the equation. Reduce until equation is similar to the standard form. Here's an example problem: 4x2 - 9y2 = 364x2 / 36 - 9y2 / 36 = 1x2 / 9 - y2 / 4 = 1x2 / 32 - y2 / 22 = 1a = 3 and b = 2Set y = 0 in the equation you got. Solve for x. The results are the x intercepts. They are both the positive and negative solutions for x. x2 / 32 = 1x2 = 32 x = ± 3 Set x = 0 in the equation you got. Solve for y and the results are the y intercepts. Remember that the solution has to be possible and a real number. If it’s not real then there is no y intercept. - y2 / 22 = 1- y2 = 22No y intercepts. The solutions aren’t real.
Solve for c and find the coordinates of the foci.See the picture for the foci equation: a and b are what you found already. When finding the square root of a positive number there are two solutions: a positive and negative since a negative times a negative is a positive. c2 = 32 + 22c2 = 5c = ± the square root of 5F1 (√5, 0) and F2 (-√5 , 0) are the fociF1 is the positive value of c used for the x coordinate along with a y coordinate of 0. (positive C, 0) Then F2 is the negative value of c that is an x coordinate and again y is 0 (negative c, 0).
Find the asymptotes by solving for the values of y. Set y = - (b/a) xand Set y = (b/a) xPlace points on a graphFind more points if needed for making a graph.
Graph the equation.The vertices are at (±3, 0). The vertices are on the x axis since the center is the origin. Use the vertices and b, which is on the y-axis, and draw a rectangle Draw the asymptotes through opposite corners of the rectangle. Then draw the hyperbola. The graph represents the equation: 4x2 - 9y2 = 36.
About the Author
Joan Reinbold is a writer, author of six books, blogs and makes videos. She has been a tutor for students, library assistant, certified dental assistant and business owner. She has lived (and gardened) on three continents, learning home renovation in the process. She received her Bachelor of Arts in 2006.