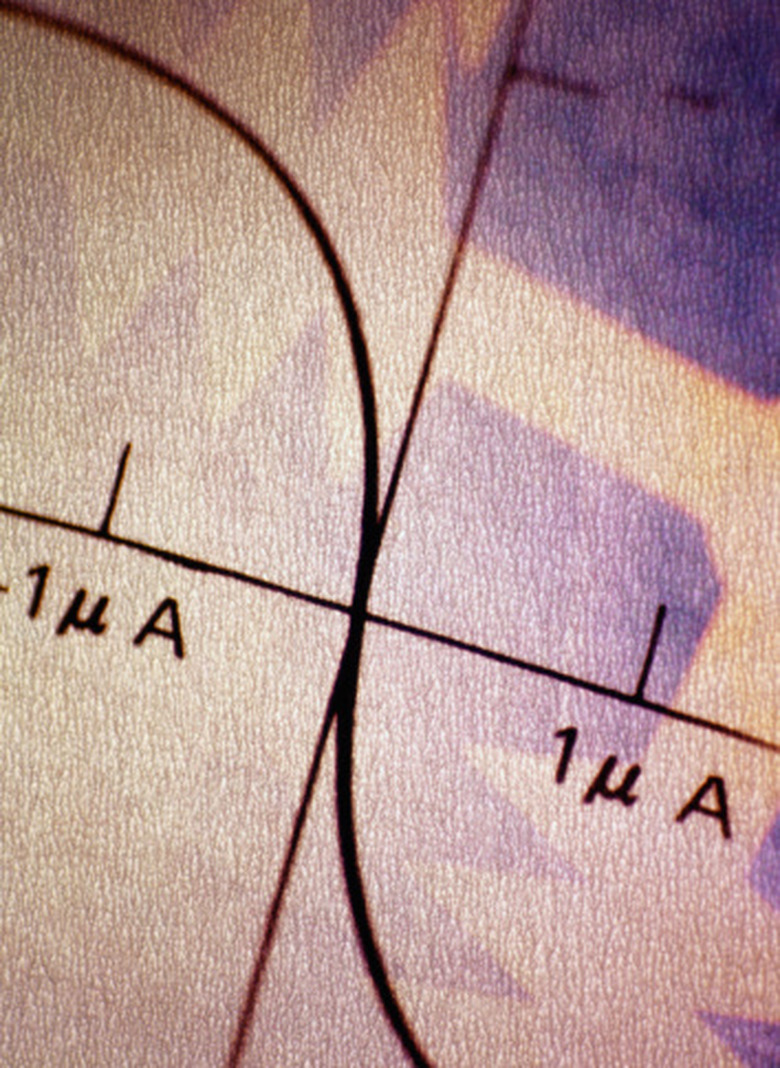
How To Find The Vertical Tangent

The vertical tangent to a curve occurs at a point where the slope is undefined (infinite). This can also be explained in terms of calculus when the derivative at a point is undefined. There are many ways to find these problematic points ranging from simple graph observation to advanced calculus and beyond, spanning multiple coordinate systems. The method used depends on the skill level and the mathematic application. The first step to any method is to analyze the given information and find any values that may cause an undefined slope.

Step 1

Observe the graph of the curve and look for any point where the curve arcs drastically up and down for a moment.

Step 2

Note the approximate "x" coordinate at these points. Use a straight edge to verify that the tangent line points straight up and down at that point.

Step 3

Test the point by plugging it into the formula (if given). If the right-hand side of the equation differs from the left-hand side (or becomes zero), then there is a vertical tangent line at that point.

Step 1

Take the derivative (implicitly or explicitly) of the formula with respect to x. Solve for y' (or dy/dx). Factor out the right-hand side.

Step 2

Set the denominator of any fractions to zero. The values at these points correspond to vertical tangents.

Step 3

Plug the point back into the original formula. If the right-hand side differs (or is zero) from the left-hand side, then a vertical tangent is confirmed.