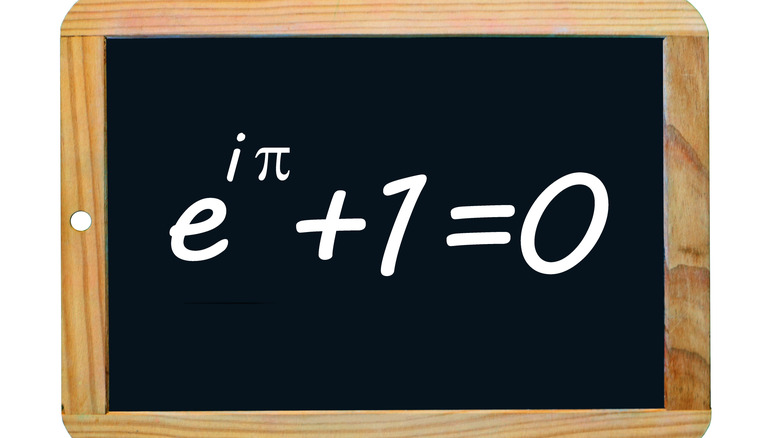Uppercase "E" stands for "exponent" in calculator displays. Calculator manufacturers use it to display numbers in scientific notation because the longhand version is difficult to display and would be even more difficult to read. To complicate matters, some calculator manufacturers use lowercase "e" to denote exponents, inviting confusion between scientific notation and Euler's number, which is a different thing altogether. Don't be taken in. If either an uppercase or lowercase letter "e" appears on your display output, it denotes an exponent. The only place you'll see Euler's number is on the keypad or as a constant that you type in.

Science is full of very large numbers and very small numbers that are difficult to read and write. For example, the mass of the earth is 5,970,000,000,000,000,000,000,000 kilograms, while the mass of a hydrogen atom is 0.00000000000000000000000000167 kilograms. Scientific notation makes these numbers easier to handle by expressing the 0's as a power of ten. This helps to condense many decimal places or multiple orders of magnitude into a smaller mathematical expression that is easier to read.

Using this notation, the mass of the Earth becomes 5.97 × 10²⁴ kg, and the mass of a hydrogen atom becomes 1.67 × 10^-27 kg. Instead of numbers with long strings of zeros that are difficult to count and even more difficult to display on a small screen, you have more manageable decimal fractions and exponents of 10.

Scientific notation works by reducing down the number to only have one number less than 10 in front of the decimal point. We do this by dividing by a certain magnitude of 10. In scientific notation, the exponent of the multiplying factor (10) will almost always be a whole number.

In its written form, scientific notation would look strange on a calculator. It would be confusing and it wouldn't fit on a small display. To avoid these problems, manufacturers created a symbol for "X 10." This symbol is either E or e, depending on the calculator. This letter is always followed by a number, which is the exponent to which 10 is raised.

On a calculator display, the mass of the earth would be shown as 5.97E24 (or 5.97e24). The number 5.97 is the argument and the number 24 is the exponent. Similarly, the mass of a hydrogen atom would be 1.67E-27 (or 1.67e-27).

Another example from pure mathematics might be taking the factorial of a number. For example, entering 20! (twenty factorial) into a calculator will likely return 2.432902E18. Indicating it is approximately 2.432902 quintillion.

It's just as difficult to punch in long strings of zeros on a calculator pad as it is to write them on paper, so calculators have a shortcut. It's the EE key. To enter a number in scientific notation, first input the argument, then press the EE key and enter the exponent. For example, to enter the mass of the earth, key in 5.97, then press the EE key and enter 24. The display will read 5.97E24 (or 5.97e24). Note that the number will appear with all its zeroes if they fit on the screen. For example, if you key in 1.2 EE 5, the display will show 120,000.

Most scientific calculators devote a special key to Euler's number, because it is one of the most important irrational numbers in mathematics and enters into all kinds of scientific calculations. This is the "e" key. Press it, and Euler's number will appear in your display to the accuracy the display allows. The scientific calculator on an iPhone, for example, shows 2.718281828459045. In addition, most calculators also have an "e^x" key. Enter a number, press this key and the display will show the value of ‌e‌ raised to the exponent you entered, as an expression of an exponential function. In both of these cases the mathematical constant ‌e‌ has a distinct meaning as the expression of scientific notation.

You can usually pick out the use cases of a number ‌e‌ compared to the letter E (as used for scientific notation) by context, or you can look for the ‘E’ to be sandwiched between two other numbers.

Euler’s constant is used for all sorts of useful calculations in the real world. It also acts as the base of natural logarithms. The natural logarithm describes a kind of inverse relationship to e (which you can read more about here). It can be briefly described with the following relationships:

Euler’s number was actually first mentioned in texts by Jacob Bernoulli and John Napier, but it was later formalized as the constant ‌e‌ by the mathematician Leonhard Euler. It is used to evaluate expressions involving complex numbers and it describes compound interest in many situations. Note that natural log can only be taken of a positive number.

Another special property of ‌e‌ is found in calculus, where the derivative of ‌e‌ is ‌e‌!